Primer on Geometric Algebra

Return to current section: Lorentz Contraction.

Exercise: Reasoning from similar triangles, derive the Lorentz contraction formula  ℓ₀ = γℓ  and discuss its physical meaning.

In the spacetime map below, the vertcal black line is the history of a lab observer who measures the length of a rod of rest length  ℓ₀ (aligned along the direction of motion) which moves with velocity v with respect to that observer. From our previous considerations of spacetime maps, the tilt of the orange lines represents the velocity and tan(β)= v/c. The rod is shown parallel to one of its lines of simultaneity. In the rest frame the ends of the rod share the same local time. In any inertial frame, lines of simultaneity are lines perpendicular to the frame's time axis (to which, in the rod's frame, the orange lines are parallel), so the distorted (in the lab frame) red right angle symbol in the diagram represents an authentic right angle in the rod's rest frame. The lab observer measures the rod as it flies past by measuring the distance ℓ from X1 to X2 (along the lab's line of simultaneity), because one end of the rod is always on the leftmost orange history line and the other end of the rod is always on the other orange history line. Several proper times are labeled in the diagram. 

We need to think more carefully about the angles mentioned in the diagram. After all, if we're planning to use similar triangle ratios, we'd better convince ourselves the important triangles really are similar. We've seen in spacetime maps and explained before that angle β is related to the relative velocity of the rod frame with respect to the lab frame. However, you may remember we've also seen that if we view things from a third frame where, for example the rod is moving forward slower and the lab is moving backwards, that angle's value changes. Still, there is one other perspective where this angle has the same value as in the diagram: the rod's rest frame, where the lab's relative velocity is - v/c. That's good enough! The angle β is the same in either the lab or rod frame.

Yet, anyone glancing at the red right angle symbol in the diagram knows immediately that, while it is logically a right angle in the rod's frame, it is certainly NOT a Euclidean right angle in the pictured lab map. We could, in fact, safely use similar triangle ratios if the sides used in ratios for the green triangle represented only lab frame values and the sides used in ratios for the pink triangle represented only rod frame values. We'd like more flexibility, though. We'd like to justify using all three triangles and ratios of any respective sides, whether those sides refer to rod frame measurements or lab frame measurements. So, what can it mean to think of the red right angle symbol as a right angle also in the lab frame? Using the spacetime displacement notation from NFCM, there is no doubt we can write for the three spacetime triangles:

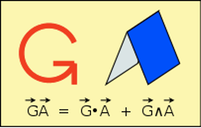
          X₂₀  ≡  X₂ - X₀  =  X₂₁ + X₁₀ ,          X₂₁  =  X₂₃ + X₃₁ ,          X₁₀  =  X₁₃ + X₃₀  

We need to be aware of the meaning of the symbols in the diagram. We label various events (such as X2), there is no ambiguity in the displacement notation of the last equations, but we're also labeling some spacetime distances in the diagram. It is ratios of these distances we'd like to equate for corresponding similar triangles. If these spacetime distances refer to the same numbers in either the rod or lab frame and we can give a meaning to the right angles which hold up in either frame, we'll be free to equate any similar triangle ratios we like. This invariance between diagram labels in the two frames will come about by writing the interval invariance expressions for the triangles. For example, for the first triangle equation above (for the green triangle):

Since the intervals above are invariants, so is the expression in the square brackets. This equation is the law of cosines for spacetime trig. The expression in the square brackets may be defined by analogy as the spacetime dot product of two spacetime displacements and is a scalar. In this diagram, the dot product becomes [(ct)( - ℓe₁) + (ℓe₁)(ct)] / 2 = 0. This is true if calculated with either the rod or lab frame coordinates. Hence both frames see the green triangle's angle near X1 to be a spacetime right angle. The same reasoning works for the red right angle in the pink triangle.    

There are actually three similar triangles in the diagram since they each have a spacetime right angle and share another angle β. These are the green triangle, the pink triangle and the large triangle made of combining the green to the pink. Interval invariance used for the three triangle equations above give helpful relationships:

These would be more than enough to prove the assertion of the exercise (use for example the second and fourth equations), but using similar triangles will get there more directly. Also, the additional practice is good and we'll be able to compare and double check relationships rediscovered. When writing ratios of related sides in the following equations, we have to be a little careful because of the visual distortions and because the direction from β towards the right angle is CW for the two smaller triangles but CCW for the large combined triangle. Here are two sets of equations using similar triangles:

The first part shows the Lorentz Contraction:  ℓ₀ = γℓ. The rod appears in the lab frame to be shorter than reported by observers in the rod's rest frame. The rod is not physically contracted, as Lorentz had proposed (compressed by motion through the ether). Rather, we're comparing measurements made by observers in relative motion. We all agree one should measure the positions of the endpoints "simultaneously" but the lines of simultaneity in one frame are not parallel to those in the other.

Below is an interactive diagram for studying the numbers in this example. You can drag the blue event X3 around to change the rod's lab coordinates and velocity relative to the lab. The coordinates were used to calculate the listed proper times. With all this data, it is easy to check any of the above relationships. Perhaps you'll spot some relationships you hadn't expected or debunk some faulty intuitions.

In this diagram, it is worth checking further examples of time dilation. The original example from a couple of sections ago is shown in the diagram as cτ₁₀ (= ct) = γ cτ₂₀. The time between ticks for the rod's clocks seems to stretch as measured on the lab's clocks. But, look at the last similar triangle ratios written above:

This has often confused students because, comparing to the red expression in the last paragraph, the relativity factor seems to be on the wrong side. Yet, this last equation is correct and also expresses time dilation. And the diagram may add to the confusion! Notice that cτ₁₀ looks smaller than cτ₂₀, yet the Lorentz Contraction formula says it's actually a bigger interval. On the other hand, cτ₁₀ also looks smaller than cτ₃₀, yet the Lorentz Contraction formula says it really is a smaller interval! Why aren't these observations in conflict? RESET the interactive diagram above by clicking in its upper right corner, so that v = 0.6 c and X3 has coordinates (pos = 6, clock = 10 on the lab map). Then check out the statements in the following paragraphs.

Reading the time for X2: The rod is in uniform motion with respect to the lab and the two have clocks reading zero when their coordinate origins coincide (for simplicity while viewing the diagram, we can temporarily assume X0 is that origin and both the rod and lab systems reset their clocks at that event). Sometime later both observe event X2. The rod frame reads the clock still resting at its origin, the proper time cτ₂₀. However X2 is no longer at the lab's origin, so the lab must read the event time along its own line of simultaneity, giving cτ₁₀. The Lorentz Contraction formula gives us the relation between these readings: cτ₁₀ = γ cτ₂₀. 

Reading the time for X1: Same setup as in the first sentence of the previous paragraph. This time, cτ₁₀ corresponds to the proper time of the clock at rest at the lab's origin. X1 is not at the rod frame's origin now, so the rod must read the event time along its own line of simultaneity, which is parallel to the rod, so we can read the rod frame's time at X3, which gives cτ₃₀. The Lorentz Contraction formula gives us the relation between these readings: cτ₃₀ = γ cτ₁₀ or cτ₁₀ = cτ₃₀ / γ.

Reading the time for X3: Same setup as in the previous paragraphs.  This time, cτ₃₀ = 4.17 corresponds to the proper time of the clock at rest at the rod's origin. X3 is not at the lab's origin, so the lab must read the event time along its own line of simultaneity, which gives ct₃₀ = ct(X3) - ct(X0) = 10 - 4.79 = 5.21. The Lorentz Contraction formula gives us the relation between these readings: ct₃₀ = γ cτ₃₀ = (1.25) (4.17) = 5.21.

Summary statement for Time Dilation:

          c∆t = time elapsed between events "not at rest" (meaning the events occur at different places in this system),
                   this time duration must therefore be read along a line of simultaneity for this system.

          c∆τ = time elapsed between events "at rest" (meaning the events occur at the same place in this system),
                   this time duration is what we've called the proper time in previous discussions.

          Time Dilation (again):    c∆t = γ c∆τ

Return to current section: Lorentz Contraction.